amateur radio
electrical
electronics
ham radio
mathematics
radio amatur
#Resistance, AC circuits, audio systems, capacitive reactance, circuit analysis, circuit design, DC circuits, electrical concepts, electrical engineering, electrical theory, electronics, engineering basics, engineering education, frequency dependence, impedance, impedance matching, inductive reactance, Ohm's Law, phase shift, power systems, reactance, RF circuits, RLC circuits, signal integrity
9M2PJU
0 Comments
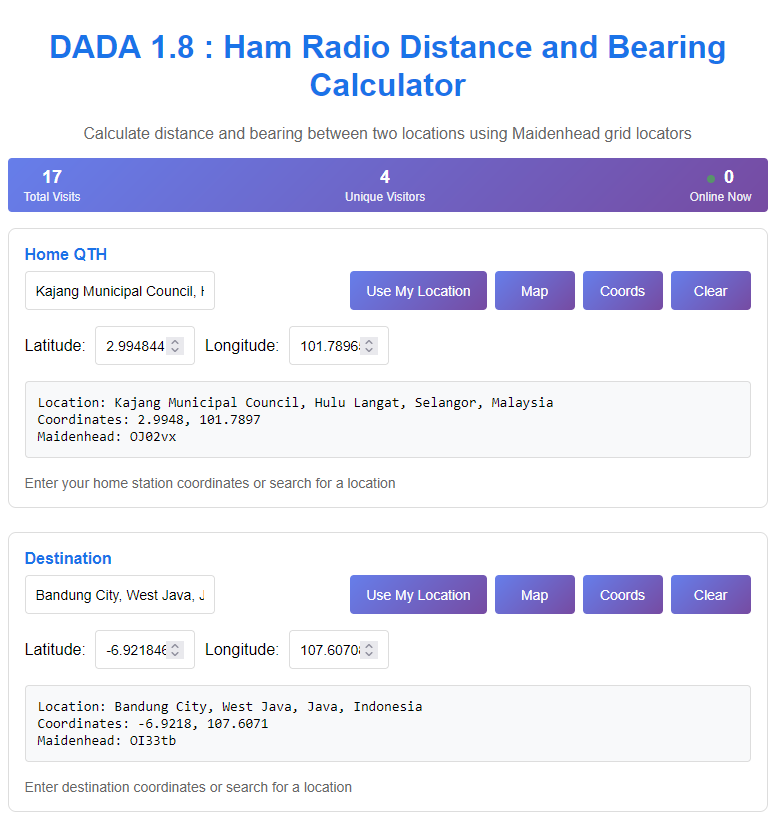
Impedance vs Resistance: A Comprehensive Guide to Understanding the Key Differences in Electrical and Electronic Systems
In the realm of electrical and electronic engineering, the concepts of resistance and impedance are foundational. While they both describe opposition to the flow of electric current, they are not interchangeable. Understanding the nuances between these two terms is critical for designing, analyzing, and troubleshooting circuits, whether you’re working with direct current (DC) or alternating current (AC) systems. This blog post will delve deeply into the differences between impedance and resistance, explore their mathematical foundations, and highlight their practical applications in real-world scenarios.
What is Resistance?
Resistance is one of the most fundamental and intuitive concepts in electrical engineering. It quantifies how much a material or component opposes the flow of electric current. Represented by the symbol R and measured in ohms (Ω), resistance is a scalar quantity that applies to DC circuits, where the current flows in a single, constant direction.
Key Characteristics of Resistance:
DC Circuits: Resistance is primarily used in DC circuits, where the voltage and current are steady and do not vary with time.
Ohm’s Law: Resistance is governed by Ohm’s Law, which states that V = I × R, where:
- V is the voltage across the component (in volts),
- I is the current flowing through it (in amperes),
- R is the resistance (in ohms).
Energy Dissipation: Resistance causes electrical energy to be converted into heat. This is why resistors are commonly used to control current and voltage levels in circuits.
Frequency Independence: Resistance is constant and does not depend on the frequency of the signal. Whether you’re working with a steady DC signal or a low-frequency AC signal, the resistance remains unchanged.
Physical Basis: Resistance arises from the collisions between electrons and the atoms in a material. Materials with high resistance (e.g., rubber) are insulators, while those with low resistance (e.g., copper) are conductors.
What is Impedance?
Impedance, represented by the symbol Z and also measured in ohms (Ω), is a more advanced concept that extends the idea of resistance to AC circuits. Unlike resistance, impedance accounts for the opposition to current flow caused not only by resistance but also by inductance and capacitance. This makes impedance a complex quantity, meaning it has both magnitude and phase.
Key Characteristics of Impedance:
AC Circuits: Impedance is primarily used in AC circuits, where the current and voltage vary sinusoidally over time. It describes how a circuit resists the flow of AC current, considering the effects of frequency.
Complex Quantity: Impedance is a combination of:
- Resistance (R): The real part of impedance, representing energy dissipation.
- Reactance (X): The imaginary part of impedance, representing energy storage and release. Reactance is further divided into:
- Inductive Reactance (Xₗ): Opposes changes in current and increases with frequency.
- Capacitive Reactance (X꜀): Opposes changes in voltage and decreases with frequency.
Frequency Dependence: Unlike resistance, impedance varies with the frequency of the AC signal. This makes impedance a critical factor in designing filters, amplifiers, and other frequency-sensitive circuits.
Phase Difference: Impedance introduces a phase difference between voltage and current in AC circuits. This phase shift is a key consideration in power systems, signal processing, and communication systems.
Physical Basis: Impedance arises from the combined effects of resistance, inductance, and capacitance in a circuit. Inductors store energy in magnetic fields, while capacitors store energy in electric fields, both of which contribute to reactance.
Impedance vs Resistance: A Detailed Comparison
To better understand the differences between impedance and resistance, let’s compare them side by side:
| Aspect | Resistance (R) | Impedance (Z) |
|---|---|---|
| Definition | Opposition to DC current | Opposition to AC current |
| Components | Pure resistance only | Resistance + Reactance (inductive/capacitive) |
| Frequency Dependence | Independent of frequency | Dependent on frequency |
| Phase Shift | No phase shift between voltage and current | Introduces phase shift |
| Mathematical Form | Scalar quantity (real number) | Complex quantity (magnitude and phase) |
| Measurement | Measured using an ohmmeter | Measured using an impedance analyzer |
| Applications | DC circuits, power dissipation | AC circuits, filters, transmission lines |
Mathematical Foundations of Impedance
Impedance is a complex quantity, meaning it has both a real part (resistance) and an imaginary part (reactance). It is typically represented in one of two forms:
Rectangular Form: Z = R + jX
- R is the resistance (real part).
- X is the reactance (imaginary part).
- j is the imaginary unit (j = √-1).
Polar Form: Z = |Z| ∠ θ
- |Z| is the magnitude of impedance, calculated as |Z| = √(R² + X²).
- θ is the phase angle, calculated as θ = tan⁻¹(X/R).
The total impedance of a circuit depends on the combination of resistive, inductive, and capacitive elements. For example:
- In a purely resistive circuit, Z = R.
- In a purely inductive circuit, Z = jXₗ.
- In a purely capacitive circuit, Z = -jX꜀.
For a series RLC circuit (resistor, inductor, and capacitor in series), the total impedance is:
Z = R + j(Xₗ – X꜀)
Where:
- Xₗ = 2πfL (inductive reactance),
- X꜀ = 1/(2πfC) (capacitive reactance),
- f is the frequency of the AC signal,
- L is the inductance,
- C is the capacitance.
Why Does the Difference Matter?
The distinction between impedance and resistance is not just theoretical—it has practical implications in various fields of electrical and electronic engineering. Here are some real-world applications where understanding impedance and resistance is crucial:
Audio Systems:
- In audio equipment, impedance matching between components (e.g., speakers and amplifiers) is critical to ensure maximum power transfer and prevent signal distortion. Mismatched impedance can lead to poor sound quality and even damage to equipment.
RF and Wireless Communication:
- In radio frequency (RF) circuits, impedance matching is vital to minimize signal reflections and ensure efficient transmission. Antennas, transmission lines, and RF amplifiers are designed with specific impedance values to optimize performance.
Power Distribution:
- In AC power systems, impedance affects the flow of current and the stability of the grid. Engineers must account for impedance when designing transformers, transmission lines, and distribution networks to minimize losses and ensure reliable operation.
Filters and Oscillators:
- Impedance plays a key role in the design of filters and oscillators, where the frequency response of the circuit is critical. For example, in a bandpass filter, the impedance of the components determines the range of frequencies that can pass through the circuit.
Signal Integrity in High-Speed Circuits:
- In high-speed digital circuits, impedance matching is essential to maintain signal integrity and prevent reflections. Printed circuit boards (PCBs) are designed with controlled impedance traces to ensure reliable data transmission.
Practical Example: Calculating Impedance in an RLC Circuit
Let’s consider a practical example to illustrate the concept of impedance. Suppose we have a series RLC circuit with the following parameters:
- Resistance (R) = 10 Ω,
- Inductance (L) = 50 mH,
- Capacitance (C) = 100 µF,
- Frequency (f) = 50 Hz.
First, we calculate the inductive and capacitive reactances:
Xₗ = 2πfL = 2π × 50 × 50 × 10⁻³ = 15.71 Ω
X꜀ = 1/(2πfC) = 1/(2π × 50 × 100 × 10⁻⁶) = 31.83 Ω
Next, we calculate the total impedance:
Z = R + j(Xₗ – X꜀) = 10 + j(15.71 – 31.83) = 10 – j16.12 Ω
The magnitude of the impedance is:
|Z| = √(R² + (Xₗ – X꜀)²) = √(10² + (-16.12)²) = 18.94 Ω
The phase angle is:
θ = tan⁻¹((Xₗ – X꜀)/R) = tan⁻¹(-16.12/10) = -58.2°
This negative phase angle indicates that the circuit is capacitive, meaning the current leads the voltage.
Conclusion
In summary, resistance and impedance are both measures of opposition to current flow, but they apply to different types of circuits and have distinct characteristics. Resistance is a straightforward concept used in DC circuits, while impedance is a more complex, frequency-dependent property essential for AC circuits. By understanding the differences between these two concepts, engineers and hobbyists can design more efficient and effective electrical and electronic systems.
Whether you’re working on a simple DC circuit or a sophisticated AC system, knowing when to consider resistance versus impedance will help you achieve better results and avoid common pitfalls. So, the next time you encounter these terms, you’ll have a clear understanding of what they mean and why they matter.





Post Comment